2.2. Kepler-Poinsot. In 1619 a first extension of the series of regular polyhedra was published by Kepler in his Harmonices Mundi. His two new regular polyhedra are not convex and are built with star-shaped faces Figure 3). About 200 years after Kepler’s publication, Poinsot found two other non-convex regular polyhedra and these were added to the family. They are shown in Figure 4.
Figure 3: Kepler Polyhedra
Figure 4: Poinsot Polyhedra
2.3. Coxeter. In 1937 Coxeter, Longuet-Higgins and Miller described three new regular polyhedra to be added to the nine previously known [3]. These infinite regular polyhedra are shown in Figures 5-7.
Figure 5: Coxeter 4-6
Figure 6: Coxeter 6-4
Figure 7: Coxeter 6-6
2.4. Infinite Polyhedra. In 1974 Wachman, Burt and Kleinmann published their book “Infinite Polyhedra” [4] that gave an introduction to and a description of the infinite uniform polyhedra. They divide the infinite polyhedra in three families: A. Cylindrical polyhedra, infinite in one direction only, B. Polyhedra with two noncollinear vectors of translation and C. Polyhedra with three noncoplanar vectors of translation. Here I will concentrate on family A.
3. Cylindrical Polyhedra

3.1. Antiprisms. Wachman, Burt and Kleinmann’s book describes six infinite groups of cylindrical polyhedra. If we do not allow two adjacent faces of a polyhedron to be coplanar then only two of their families are left: the family of polyhedra composed of antiprismatic rings and the group composed of helicoidal strips of equilateral triangles. In this publication the authors classify these polyhedra as regular uniform polyhedra.

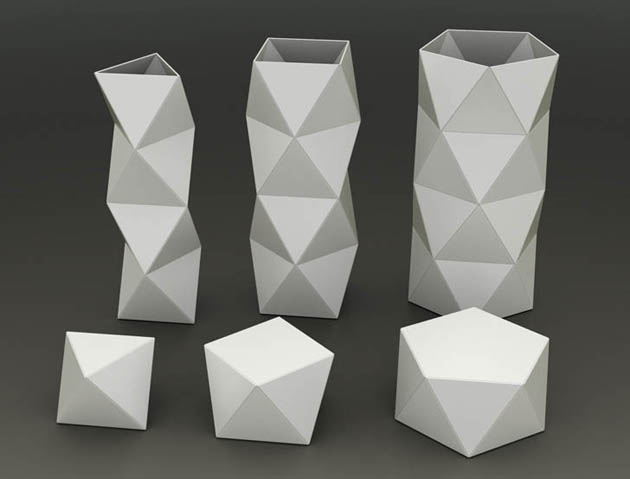
Figure 8: Infinite Polyhedra composed of Antiprismatic Rings
3.2. Tetrahelix. The tetrahelix can be seen as assemblage of tetrahedra and it is also the first member of the family of polyhedra composed of helicoidal strips. It can be unfolded as in Figure 11 in three side-by-side connected strips of equilateral triangles. In general, the polyhedra of this family, which we will call helical deltahedra, can be unfolded into a series of side-by-side connected strips.
Figure 9: Tetrahedra
Figure 10: Tetrahelix
Figure 11: Unfolding