7. Vertex Figures

7.1. Vertex Figure. When we look at the vertex figure of the regular helical star deltahedra shown so far we see that it is a non-convex hexagon without intersections (Figure 44a).
Figure 44a: Vertex Figure without Intersection
Figure 44b: Vertex Figure with Intersection
After seeing this I investigated the possibility of constructing regular helical star deltahedra which have vertex figures with intersections as in Figure 44b. It turned out to be possible and so far I have constructed a first set of 12 different regular helical star deltahedra with vertex figures of the type of Figure 44b.
7.2. Examples. Two examples of this second group are shown in Figures 45 and 46.
Figure 45: Example 1
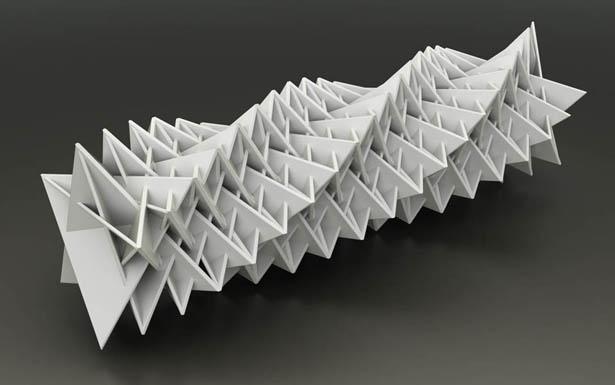
Figure 46: Example 2
Classification of members of this group is more complicated. We we can construct these polyhedra with the same folding technique as in section 4.1. This will be one of the subjects for further research. The computer program Rhinoceros and especially the plug-in Grasshopper, developed by David Rutten, were a great help in this research project.
7.3. Further Research. A more detailed version of the paper is being prepared for publication in an appropriate journal.

References

[1] Branko Grünbaum, An enduring error, University of Washington, 2008.
[2] H.S.M. Coxeter, M.S. Longuet-Higgins and J.C.P. Miller, Uniform Polyhedra, Philos. Trans. Roy. Soc. London, 1953/54.
[3] H.S.M. Coxeter, Regular Skew Polyhedra in Three and Four Dimensions, Proc. London Math. Soc. 43, 33-62, 1937.
[4] A. Wachman, M. Burt and M. Kleinmann, Infinite Polyhedra, Technion, Haifa, 1974.
[5] David Rutten, Grasshopper, www.grasshopper3d.com.