4. Weaving Patterns on Polyhedra

4.1. Rings on the Sphere. In the group of regular and semi regular polyhedra there are, except from the anti prisms, five polyhedra that can be colored in a checkerboard fashion. And they all have vertices with degree 4. This gives us five weave patterns on the sphere as can be seen in Figure 14.
In Japanese Temari Balls and also in Alan Holden’s Orderly Tangles [3] we can find many examples of the use of these basic patterns.
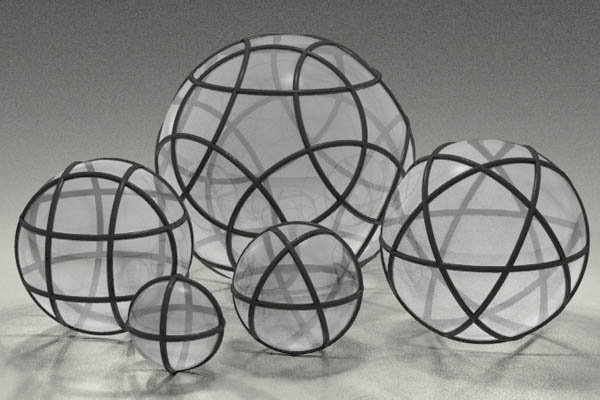
Figure 14: Five basic Patterns
But with the procedure described in section 3.1 we can also derive weave patterns from the other Platonic and Archimedean solids. In Figures 15 to 20 some examples are shown. In the weavings we still get closed loops but these lines are not laying in a cutting plane of the polyhedron as in the first five. And in some cases (snub cube and snub dodecahedron), the loops go around twice, crossing themselves a few times.
The weavings shown in Figures 15 – 20 are based on the Rhombic Truncated Cuboctahedron, the Truncated Dodecahedron, the Truncated Dodecahedron, the Rhombic Truncated Icosidodecahedron, the Snubcube and the Snubdodecahedron.
Figure 15: Rhombic Truncated Cuboctahedron
Figure 16: Truncated Dodecahedron
Figure 17: Truncated Dodecahedron
Figure 18: Rhombic Truncated Icosidodecahedron
Figure 19: Snubcube
Figure 20: Snubdodecahedron