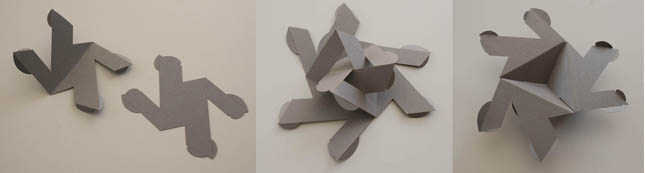
To start building the model of the edge-elevated icosahedron I first connect three pairs of faces as you can see in Figure 19. Both the top and the bottom are shown.
Figure 19:
Construction elements – Three connected elements (top view) – Three connected elements (bottom view).
As you can see, when you connect more elements, the structure at the outside becomes quite complicated, while the inside of the polyhedron is a nice diamond pattern.
Figure 20ab: Ten pairs of faces are connected.
Figure 20cd: Twenty pairs of faces.
5. Examples

5.1. Geodesic Spheres. The method of elevating the midpoint of each of the edges of a polyhedron can be applied on many different objects. For the examples I have limited myself to objects built with only triangles. The triangles don’t have to be equilateral, as can be seen in the examples of the geodesic spheres in Figure 21 and Figure 22. Because the construction is double layered, it is quite strong and could very well be used for dome constructions.
Figure 21a: Geodesic sphere - inside.
Figure 21b: Geodesic sphere - outside.
Figure 22a: Geodesic sphere.
Figure 22b: Half a sphere, dome
5.2. Cylinders. TAs a final example I would like to show a cylindrical construction. The basic polyhedron is a helical deltahedra. I think we may conclude that the “edge elevation” is an interesting new operation with which we can generate interesting constructions.
Figure 23: Edge elevated cylinder.

References

[1] Luca Pacioli, La Divina Proportione, Edicione Akal, Madrid, 1991 (first published in 1509).
[2] Luca Pacioli, Divina Proportione: Die Lehre Vom Goldenen Schnitt, 1509, Ed. Carl Graeser, Wien, 1896.
[3] Peter R. Cromwell, Polyhedra, Cambridge University Press, UK, 1997.
[4] Red. J.W. Vermeulen, Het oneindige, M.C. Escher over eigen werk, Meulenhoff, Amsterdam, 1986.
[5] Magnus J. Wenninger, Polyhedron Models, Cambridge University Press, UK, 1971.