 |
 |
 |
 |
 |
 |
 |
|
|
|
|
 |
|
|
 |
|
|
5. Connecting Holes
5.1. Reducing the number of knots. We have seen that we can reduce the number of knots by connecting them. Let us go back to the Pacioli’s elevation of the cube. We will take the version with the alternative order of the faces around the cube vertices, the “double cube” of Figure 20.
|
|
|
 |
|
|
 |
|
 |
|
 |
|
|
Figure 31: Connected elevated Cubes.
|
Figure 32: RP-model.
|
Figure 33: Connected Cubes rounded.
|
|
|
We can now connect two of these double cubes by removing one of the pyramids plus the underlying face of both of the elevated cubes (Figure 31). Around the new vertices where two double cubes get connected, the edge of the holes are now changed into sets of pairs of intertwined loops, since the valance of the connecting vertices between two plain cubes is now 4. To make this more visible we can round off all the pyramids. In Figure 32 this is done for the single elevated cube and we can see the knot-shape holes at the vertices In Figure 33, the rounded of version of the connected elevated cubes, and now we see the linked loop-shape holes in the middle. |
|
|
| 5.2. Linked Cubes. The rounded versions of the elevated polyhedra are in fact polyhedra in which each face is doubled. The single rounded version of the elevated cube has 2 x 6 “square” faces. The connected elevated cube model has 2 x 10 “square” faces. In the vertices in the middle of the object in Figure 33 we see 2 x 4 faces coming together and in the other vertices 2 x 3 faces are coming together. The odd number 3 leads to a knot-shaped hole, whereas the even number 4 leads to the two entwined simple loop-shaped holes. |
|
| 5.3. Linked Squares. Looking at the connection of 4 elevated cubes (Figure 34), we can think of other applying the “doubling transformation” on other patterns with squares. |
|
 |
|
 |
|
 |
|
|
Figure 34: 4 Connected Cubes.
|
Figure 35: Square Pattern.
|
Figure 36: Elevated Squares.
|
|
| The cube is the regular polyhedron build with squares. In the plane we have the regular tiling with squares (Figure 35). Also here we can “double” each of the squares to make a construction similar to the idea of Elevation. The elevation of this pattern, or the “doubled square pattern” is shown in Figure 36. As we can see, this structure falls apart into two entwined layers, so it is a compound. |
|
|
6. Coxeter’s infinite Polyhedra
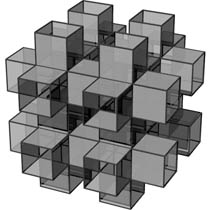
6.1. Elevation of the Coxeter’s infinite polyhedron 444444. Beside the cube and the regular square tiling there is one other regular structure build with squares that can be studied in this context. This is Coxeter’s infinite polyhedron 444444 (Figure 37), in which 6 squares meet at each vertex.
|
|
 |
|
 |
|
 |
|
|
Figure 37: Coxeter 444444.
|
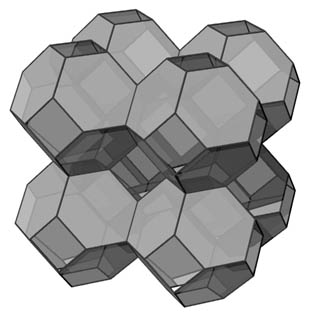
Figure 38: Elevated 444444.
|
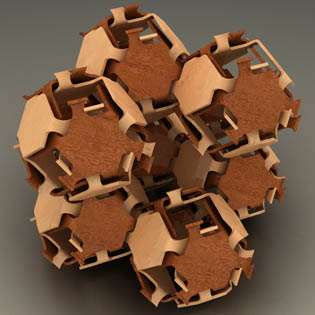
Figure 39: Around the Vertex.
|
|
| It turns out to be possible to make a “double” version of this infinite polyhedron as can be seen in Figure 38. The situation around each of the vertices can be described as the entwinement of two simple loops (Figure 39). I think we can see the structure in Figure 38 as the elevation of Coxeters polyhedron 444444. And this elevation is a compound, two separate structures, entwined in space. |
|
|
|
| 6.2. Elevation or “Doubling the Faces”. The technique we applied to create the “double” version of Coxeter’s polyhedron 444444 can be seen as a generalization of the Elevation operation. In Figure 41 the “double” version of Coxeter’s polyhedron 6666 (Figure 40) is shown. |
|
 |
|
 |
|
|
Figure 40: Coxeter 6666.
|
Figure 41: Elevated Coxeter 6666.
|
|
| 6.3. Conclusion. With the introduction of Elevation by Luca Pacioli and Leonardo da Vinci the interesting field of double layer structures has been opened. This paper is just meant to be an introduction to some more creative possibilities of this “new” concept, in addition to two former papers about this subject [4] and [5]. And still there is a lot to be investigated. |
|
|
|
References
[1] Luca Pacioli – Leonardo da Vinci, La Divina Proportione, 1509, Ed. Akal, S.A., Madrid, 1991
[2] Luca Pacioli, Divina Proportione: Die Lehre Vom Goldenen Schnitt, 1509, Ed. Carl Graeser, Wien, 1896.
[3] Rinus Roelofs, Connected Holes, Bridges Proceedings, Leeuwarden, 2008.
[4] Rinus Roelofs, Elevations and Stellations, Bridges Proceedings, Seoul, 2014.
[5] Rinus Roelofs, The Concept of Elevation applied on Flat Patterns, Bridges Proceedings, Baltimore, 2015.
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|










