1.2. Escher's intention. Based on the fact that Escher wrote "A: .." in the title we may assume that he intended to go on with another display to be called "B: ..". The next one would certainly be based upon a triangular grid because the first sketches for the display, numbered 1, 2 and 3 (Figure 2) are in the same notebook.
But besides that, Escher might have had a special reason for studying this group of patterns. For between the pages of the notebook there were a few sheets with sketches which were made as a preparation for a stamp that Escher designed for the Wereldpostvereniging, Holland (1949). On the stamp we can see a sphere decorated with entwined circular rings. So I think that besides the possibilities of using patterns with entwined circular rings in the plane, Escher was also interested in the more complex problem: which patterns can be made on three dimensional objects like the sphere?
Figure 3: The decorated sphere on the stamp.

2. From the plane to the sphere

2.1. Cube. The two infinite structures in Figure 3 and Figure 5 are both generated by doubling the basic pieces. A first idea in trying to find new shapes and structures was by introducing a 'folding' line into the pieces. Not as a hinge between the two 'half' pieces but as a rigid connection between the parts. In the first example with the basic structure of the ring of Figure 2 (three slide directions) the use of the 'folded' pieces leads to an object that looks like 2 connected cubes. And also this object can only be taken apart in two groups of three pieces before you can separate all the pieces from each other.
Figure 4: Escher's sketch
Figure 5: A cube decorated with Escher's pattern

So if going from 2D to 3D was an underlying reason, it is even more obvious that the next step in his 'Entwined Circular Ring'-project was to make a display of entwined circular ring patterns based on a triangular grid. Because from a triangular grid we can not only go to tetrahedron, but also to an octahedron, icosahedron or in general to deltahedra, including the infinite structures.

2.2. Cube and octahedron. In space there is an easy way to go from squares to triangles: the dual of the cube is the octahedron. Escher was very familiar with the relationship between the cube and the octahedron. He made several drawings of this combination and one of them is on the page in the notebook with the three circular patterns based on the triangular grid. And in the sketches for the stamp we can find some studies of using the pattern based on a triangular grid in order to be able to use the octahedron as the step in between to come to the decorated sphere (Figure 7).
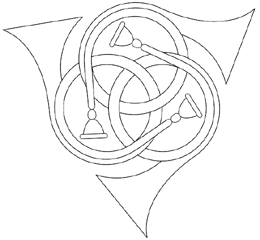
Figure 6: Cube and Octahedron
Figure 7: Escher's triangular pattern