3. Interwoven Surfaces

3.1. Regular and semiregular tilings. The underlying pattern of the structure in Figure 10 is the regular tiling 6,6,6. The structure in Figure 12 has the semiregular (or Archimedean) tiling 4,3,4,3,3 as the underlying pattern. These tilings are composed of regular polygons such that at the vertices of each tile you will find the same configuration of regular polygons. And therefore the same number of tiles meet at each vertex. For the structure in Figure 10 this number is three, which is an odd number. And because this is an odd number we will get a knot figure as a hole in our interwoven layer structure (see Figure 8c). When this number is even we will get a combination of two or more rings (see Figure 8b).
At each vertex of the underlying pattern of the structure in Figure 12 we have 5 polygons (going around a vertex we see a square, a triangle, a square, and two triangles). Also in this tiling the number of polygons in each vertex is odd and so the resulting interwoven layer structure will again be a single surface, because of the knot-shaped holes. Other such surfaces are shown in Figures 13, 14, and 15.
Figure 13: Tiling 6,6,6
Figure 14: Tiling 8,8,4
Figure 15: Tiling 6,3,3,3,3
3.2. Dual grids. Starting with the Archimedean tilings we can generate another group of tilings, the so- called ‘dual tilings’. When we connect the midpoint of each tile with the midpoints of its surrounding tiles we get a new pattern, the dual pattern of the tiling. In the dual tiling, all tiles are congruent. In Figure 16 this is demonstrated for the Archimedean tiling 4,3,4,3,3. Figures 17 and 18 show the interwoven structure and its dual. Figures 19-21 show additional dual interwoven structures. And again when odd numbers occur as in the number of tiles in a vertex of the tiling we will get interwoven structures built out of one single surface.
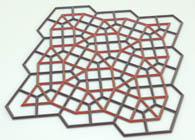
Figure 16a: Tiling 4,3,4,3,3
Figure 16b: Creating the dual
Figure 16c: Dual of 4,3,4,3,3
Figure 17: Base: tiling 4,3,4,3,3
Figure 18: Base: dual of 4,3,4,3,3
Figure 19: Base: dual of 6,3,3,3,3
Figure 20: Base: dual of 6,3,6,3
Figure 21: Base: dual of 6,4,3,4